Marcial Gonzalez

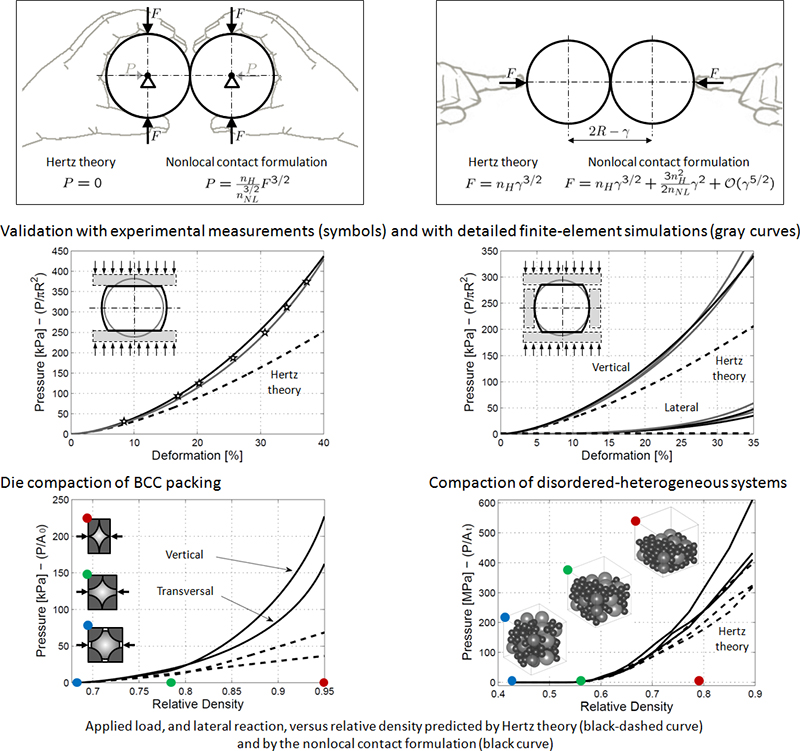
We present a nonlocal formulation of contact mechanics that accounts for the interplay of deformations due to multiple contact forces acting on a single particle. The analytical formulation considers the effects of nonlocal mesoscopic deformations characteristic of confined granular systems and, therefore, removes the classical restriction of independent contacts. This is in sharp contrast to traditional contact mechanics theories, which are strictly local and assume that contacts are independent regardless the confinement of the particles. For definiteness, we restrict attention to elastic spheres in the absence of gravitational forces, adhesion or friction. Hence, a notable feature of the nonlocal formulation is that, when nonlocal effects are neglected, it reduces to Hertz theory. Furthermore, we show that, under the preceding assumptions and up to moderate macroscopic deformations, the predictions of the nonlocal contact formulation are in remarkable agreement with detailed finite-element simulations and experimental observations, and in large disagreement with Hertz theory predictions---supporting that the assumption of independent contacts only holds for small deformations. The discrepancy between the extended theory presented in this work and Hertz theory is borne out by studying periodic homogeneous systems and disordered heterogeneous systems.